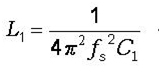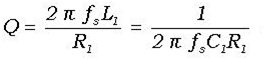The frequency of a crystal resonator is typically specified in megahertz (MHz) or kilohertz (kHz). The normal frequency is the output frequency what we expect from the crystal oscillation circuitry with proper matching. There is an amount of frequency deviation from the nominal frequency at ambient temperature (referenced to 25oC) for a real device. The tolerance of the idea central frequency deviation, as a parameter of the device, is specified with a maximum value, expressed in percent (%) or parts per million (ppm).

(2) Fundamental and Overtone Vibrations

The thickness shear vibration is the main vibration mode existed in AT cut. The high order harmonic vibrations are co-existed with fundamental vibration between electrode areas. Due to the reverse polarity of two electrodes, only odd number harmonic vibrations can be excited in piezoelectric quartz resonators.

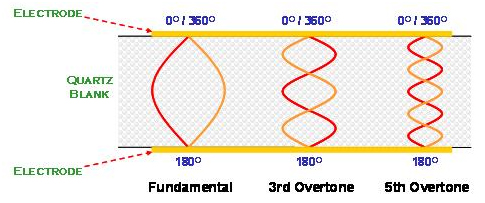
(Fig.1) Only odd number harmonic vibrations can be excited in crystal resonator

(3) Load Capacitance

Load capacitance, CL, is the amount of capacitance that the oscillator exhibits when looking into the circuit through the two ends of the resonator. The load capacitance is formally in either series or parallel with the resonator. For parallel load case the existence of CL will affect the parallel resonance frequency and the parallel-load resonance frequency, fL, is given by

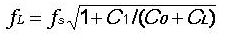
This parameter is necessary to be specified.

(4) Frequency-Temperature Stability

Frequency-Temperature stability is indicated by the amount of frequency variation from the value at the standard amblient temperature (25oC, usually), caused by the operating temperature change. This parameter is specified by a curve showing the frequency variation (expressed in % or ppm) versus the temperature deviation from the reference temperature (25oC). The temperature stability of a quartz device depends on the type of cut, the mode of vibration, and the dimension of the quartz blank. Besides, the deviation value is associated with the operating temperature range, the load capacitance and the drive level of the cryatal resonator.

(5) Equivalent Series Resistance (ESR)

The resistance R1 appearing in the series branch (fig. 5) can be measured at series resonance frequency, where the effects of C1 and L1 are cancelled each other and the effective result of the branch is a resistive. R1 represents the mechanical loss in the crystal unit and the holder.

(6) Motional Capacitance C1 and Motional Inductance L1

These two parameters are definitely related by the series resonance frequency, fs, as given in Eq.(1), and fs is a very sure parameter in resonator design and in characterization. Only the value of C1 is specified in industry standard and L1 can be obtained from

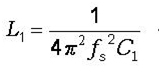
The value of C1 is very small in comparison with capacitances usually used in oscillation circuits and can be evaluated from the material and geometry parameters of the crystal plate and electrodes.

(7) Static Capacitance Co (in Shunt)

The shunt capacitance, Co , is a static capacitance, which is present whether the device is oscillating or not. The value of Co can be measured at very low frequency (less than or about 1.0 MHz), and theoretically is given by

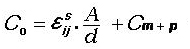
where, A is the electrode area, d is the thickness of the blank, and ε is the dielectric constant of the corresponding crystal cut.

In practically, Co includes not only the static capacitance of plated quartz blank, but also the capacitance of conductive bonding material and the capacitance of housing itself.

(8) Drive Level

The drive level of a resonator is the amount of power dissipation, expressed in nanowatts, microwatts or milliwatts. Operating level is the suitable power range to assure proper start and maintain a steady state oscillation. Drive level should be operated at the minimum level to avoid long-term frequency drift and crystal fracture. Generally, the smaller the product is, the lower the drive level should be applied without damanging the quartz resonators for long term usage. Generally, the drive level from 10μW to 100 μW is good enough for most of the applications.

(9) Quality Factor-Q

As a resonator, quality factor-Q value is a very important parameter. In specification, unloaded and loaded Q values are specified. The unloaded Q, or mechanical Q, can be expressed by

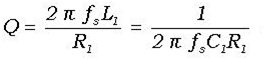
where, R1 is the resistance appearing in the series branch.
The loaded Q value depends on the loaded circuit.

(10) Pullability

In a parallel-load capacitance oscillator, the oscillation frequency depends on the load capacitance, CL as shown in Eq.(3) and (Fig. 2). The frequency change (in ppm) as a function of the load capacitance change (in pF) is a specification. In certain applications where the variation of loaded resonance frequency is mandatory (VCXO, for example), pullability has to be specified.

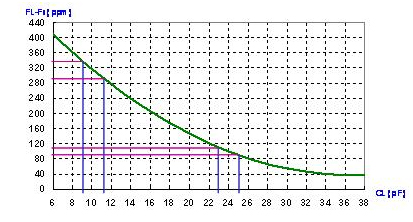
(Fig. 2) Frequency variation vs. load capacitance

(11) AGING

Aging is the relative change of operating frequency over a specified time period and is expressed in parts per million (ppm) within a specified period. This rate of frequency change is normally exponential in character. The highest aging rate occurs within the first week of aging and decreases slowly after wards. Typically, aging is computed within first 30 days and is calculated over a long-term period (one year or ten years). Aging rate depends on many factors: seal method, integrity, manufacturing processes, material type, operating temperature, and frequency.

(12) STORAGE TEMPERATURE RANGE

The specification indicates the minimum and maximum temperatures in which the devices can be stored or exposed in a non-operating state. After storing or exposing the devices at the specified temperature range for a long time, all of the specifications are guaranteed over the specified operating temperature range.

(13) Negative Resistance "-R"

resonator. This is an important parmeter in designing oscillators.

Negative resistance is introduced to describe the electric property of an oscillator circuit, This is the amount of resistance that the oscillator circuit exhibits when looking into the circuit through the terminals of the resonator. One of the basic oscillation conditions demands the amplifier have to supply enough gain to compensate the loss in the resonator. From resonator point of view, the load has to exhibit enough "negative resistance" to compensate the resistance of the resonator. This is an important parmeter in designing oscillators.